BTN.com staff, July 27, 2015
Anyone who?s commuted in traffic in a major U.S. city or tried to get their children out the door for school can claim to have some familiarity with the concept of ?controlled chaos.? Simply put, this phrase refers to the often random, unpredictable interactions and events that can hinder or even halt complex, critical systems.

Indiana University professor and researcher Filippo Radicchi recently devised a mathematical framework to analyze these scenarios. His approach is designed to improve the resilience of intricate systems such as air traffic control networks and power grids by figuring out how they might break down before they actually do.
Though he mainly uses his equations to investigate infrastructure, Radicchi said it can also be used with other complex systems, such as the human body, epidemics and social networks.
?The same kind of framework can be applied to many, many different domains,? he said.
(And here's an interesting side note: Radicchi once applied his mathematical mind to figuring out the best professional men's tennis players of all time. Who was at the top of his list, you ask? None other than the spirit of East St. Louis, Jimmy Connors.)

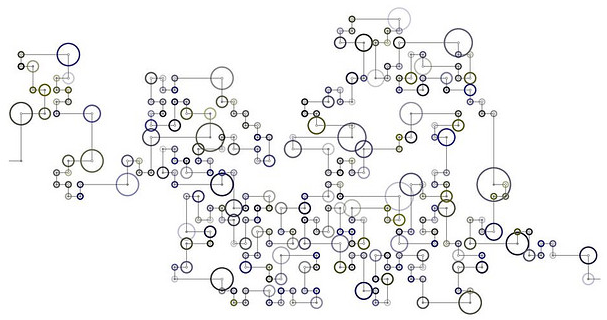
Radicchi?s equations are designed to untangle multiple complex systems by pulling apart each network - or ?graph? - for individual analysis, then reconstructing an overall picture. A ?graph? describes the multitude of points and connection lines that comprise a complex network.
Separating multiple graphs gives Radicchi and other researchers a more complete idea as to their interdependence and isolation within a network. It also provides them an opportunity to figure out different types of constraints within a system - for instance, the cost of running a cable from one generator to another within a power grid.
?You can include many factors [in order to] optimize the functionality and robustness of a system,? Radicchi said.
Radicchi?s formulas have two main advantages over prior methods of analyzing complex systems. First, they don?t require time- and cost-intensive simulations. Also, they can quickly and effectively gauge the ?percolation? in a system, meaning the amount of disruption caused by small breakdowns.
[btn-post-package]These equations would have come in handy in Radicchi?s native country of Italy back in 2003, when the nation?s power grid failed within a matter of minutes, resulting in a massive, sustained blackout. The cause of this catastrophic event was the fact that the nation?s generators were dependent on a telecommunications network that itself would be shut down by any stoppage of electrical power. This produced a cascading effect between the two different networks.
Radicchi said he and other researchers will continue to improve on this framework and develop better modeling and algorithms. And who knows? Someday it might even help the kids get to school more quickly.
By Maggie Hays